Images: The World's Most Beautiful Equations
Numerical beauty

Mathematical equations aren't just useful — many are quite beautiful. And many scientists admit they are often fond of particular formulas not just for their function, but for their form, and the simple, poetic truths they contain.
While certain famous equations, such as Albert Einstein's E = mc^2, hog most of the public glory, many less familiar formulas have their champions among scientists. LiveScience asked physicists, astronomers and mathematicians for their favorite equations; here's what we found:
General relativity
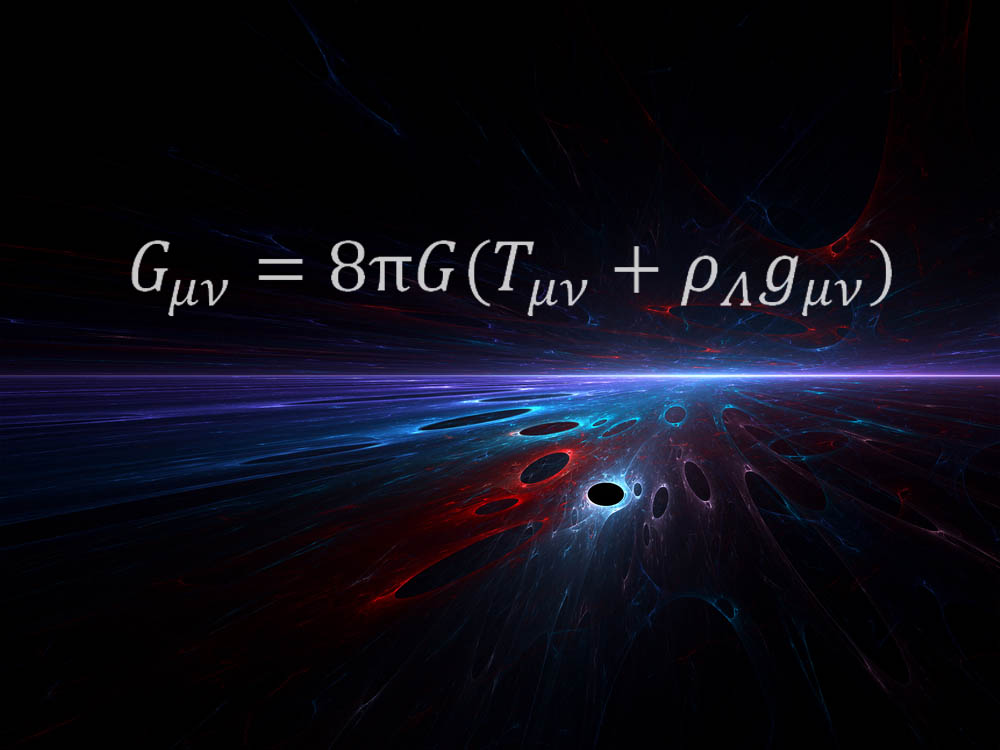
The equation above was formulated by Einstein as part of his groundbreaking general theory of relativity in 1915. The theory revolutionized how scientists understood gravity by describing the force as a warping of the fabric of space and time.
"It is still amazing to me that one such mathematical equation can describe what space-time is all about," said Space Telescope Science Institute astrophysicist Mario Livio, who nominated the equation as his favorite. "All of Einstein's true genius is embodied in this equation." [Einstein Quiz: Test Your Knowledge of the Genius]
"The right-hand side of this equation describes the energy contents of our universe (including the 'dark energy' that propels the current cosmic acceleration)," Livio explained. "The left-hand side describes the geometry of space-time. The equality reflects the fact that in Einstein's general relativity, mass and energy determine the geometry, and concomitantly the curvature, which is a manifestation of what we call gravity." [6 Weird Facts About Gravity]
"It's a very elegant equation," said Kyle Cranmer, a physicist at New York University, adding that the equation reveals the relationship between space-time and matter and energy. "This equation tells you how they are related — how the presence of the sun warps space-time so that the Earth moves around it in orbit, etc. It also tells you how the universe evolved since the Big Bang and predicts that there should be black holes."
Standard model

Another of physics' reigning theories, the standard model describes the collection of fundamental particles currently thought to make up our universe.
The theory can be encapsulated in a main equation called the standard model Lagrangian (named after the 18th-century French mathematician and astronomer Joseph Louis Lagrange), which was chosen by theoretical physicist Lance Dixon of the SLAC National Accelerator Laboratory in California as his favorite formula.
"It has successfully described all elementary particles and forces that we've observed in the laboratory to date — except gravity," Dixon told LiveScience. "That includes, of course, the recently discovered Higgs(like) boson, phi in the formula. It is fully self-consistent with quantum mechanics and special relativity."
The standard model theory has not yet, however, been united with general relativity, which is why it cannot describe gravity. [Infographic: The Standard Model Explained]
Calculus
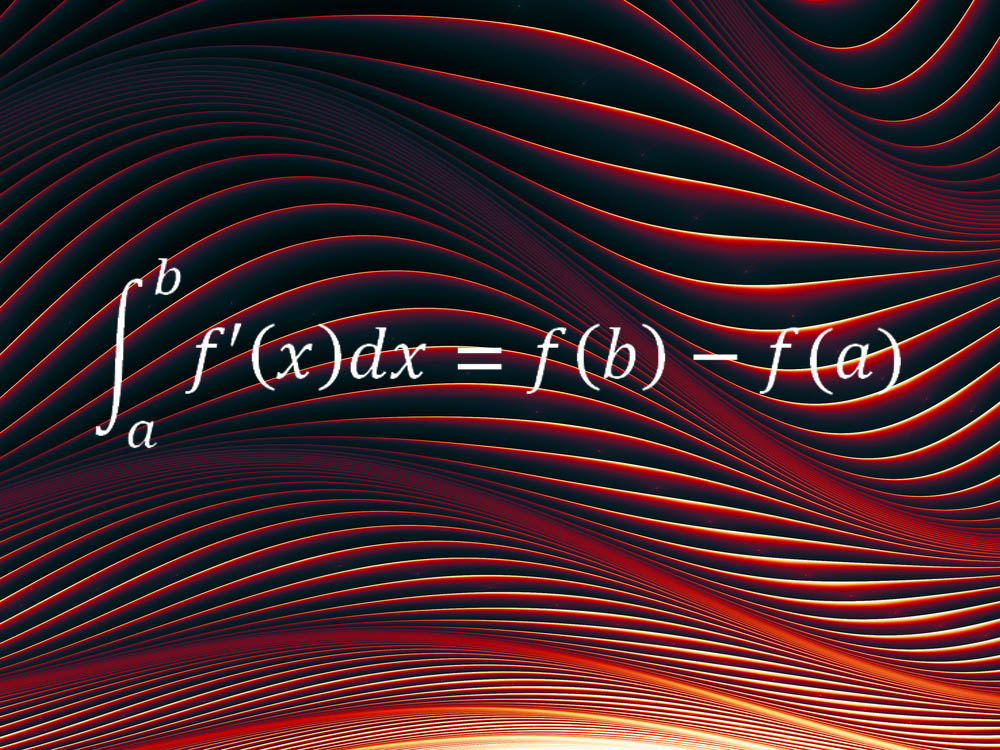
While the first two equations describe particular aspects of our universe, another favorite equation can be applied to all manner of situations. The fundamental theorem of calculus forms the backbone of the mathematical method known as calculus, and links its two main ideas, the concept of the integral and the concept of the derivative.
"In simple words, [it] says that the net change of a smooth and continuous quantity, such as a distance travelled, over a given time interval (i.e. the difference in the values of the quantity at the end points of the time interval) is equal to the integral of the rate of change of that quantity, i.e. the integral of the velocity," said Melkana Brakalova-Trevithick, chair of the math department at Fordham University, who chose this equation as her favorite. "The fundamental theorem of calculus (FTC) allows us to determine the net change over an interval based on the rate of change over the entire interval."
The seeds of calculus began in ancient times, but much of it was put together in the 17th century by Isaac Newton, who used calculus to describe the motions of the planets around the sun.
Pythagorean theorem

An "oldie but goodie" equation is the famous Pythagorean theorem, which every beginning geometry student learns.
This formula describes how, for any right-angled triangle, the square of the length of the hypotenuse (the longest side of a right triangle) equals the sum of the squares of the lengths of the other two sides.
"The very first mathematical fact that amazed me was Pythagorean theorem," said mathematician Daina Taimina of Cornell University. "I was a child then and it seemed to me so amazing that it works in geometry and it works with numbers!" [5 Seriously Mind-Boggling Math Facts]
Euler's equation
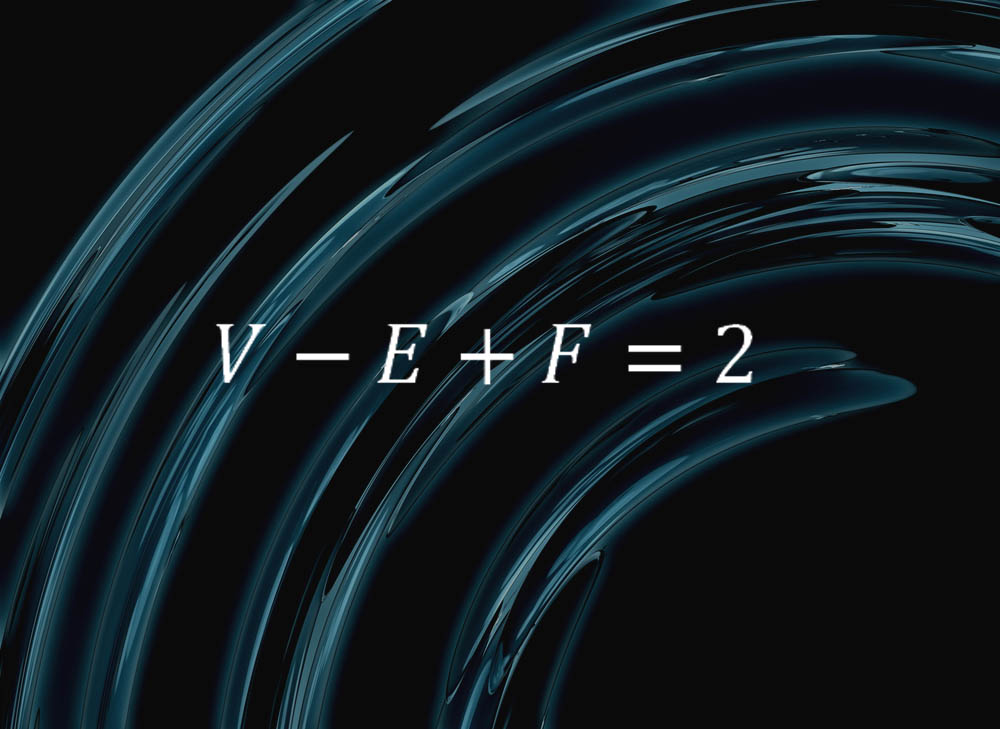
This simple formula encapsulates something pure about the nature of spheres:
"It says that if you cut the surface of a sphere up into faces, edges and vertices, and let F be the number of faces, E the number of edges and V the number of vertices, you will always get V – E + F = 2," said Colin Adams, a mathematician at Williams College in Massachusetts.
"So, for example, take a tetrahedron, consisting of four triangles, six edges and four vertices," Adams explained. "If you blew hard into a tetrahedron with flexible faces, you could round it off into a sphere, so in that sense, a sphere can be cut into four faces, six edges and four vertices. And we see that V – E + F = 2. Same holds for a pyramid with five faces — four triangular, and one square — eight edges and five vertices," and any other combination of faces, edges and vertices.
"A very cool fact! The combinatorics of the vertices, edges and faces is capturing something very fundamental about the shape of a sphere," Adams said.
Special relativity
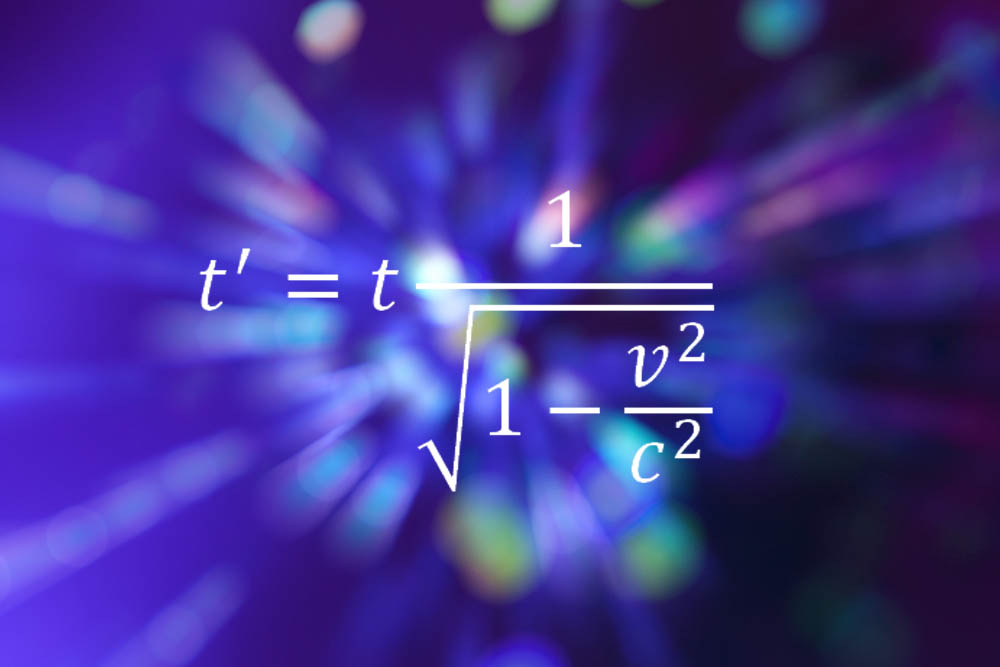
Einstein makes the list again with his formulas for special relativity, which describes how time and space aren't absolute concepts, but rather are relative depending on the speed of the observer. The equation above shows how time dilates, or slows down, the faster a person is moving in any direction.
"The point is it's really very simple," said Bill Murray, a particle physicist at the CERN laboratory in Geneva. "There is nothing there an A-level student cannot do, no complex derivatives and trace algebras. But what it embodies is a whole new way of looking at the world, a whole attitude to reality and our relationship to it. Suddenly, the rigid unchanging cosmos is swept away and replaced with a personal world, related to what you observe. You move from being outside the universe, looking down, to one of the components inside it. But the concepts and the maths can be grasped by anyone that wants to."
Murray said he preferred the special relativity equations to the more complicated formulas in Einstein's later theory. "I could never follow the maths of general relativity," he said.
Sign up for the Live Science daily newsletter now
Get the world’s most fascinating discoveries delivered straight to your inbox.
1 = 0.999999999….

This simple equation, which states that the quantity 0.999, followed by an infinite string of nines, is equivalent to one, is the favorite of mathematician Steven Strogatz of Cornell University.
"I love how simple it is — everyone understands what it says — yet how provocative it is," Strogatz said. "Many people don't believe it could be true. It's also beautifully balanced. The left side represents the beginning of mathematics; the right side represents the mysteries of infinity."
Euler–Lagrange equations and Noether's theorem
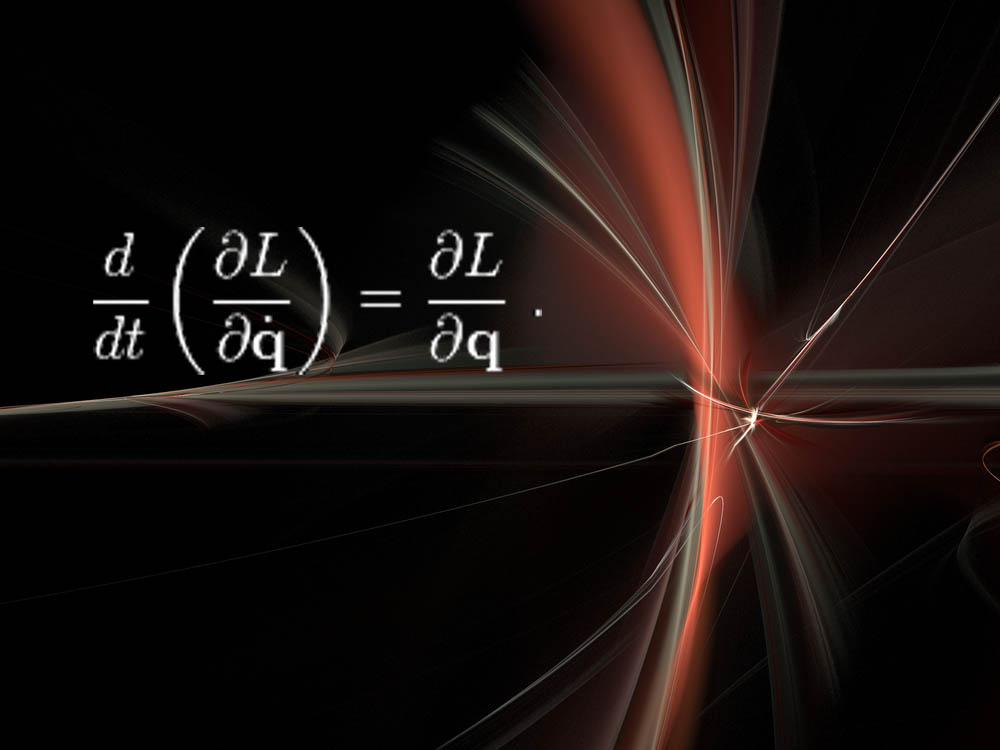
"These are pretty abstract, but amazingly powerful," NYU's Cranmer said. "The cool thing is that this way of thinking about physics has survived some major revolutions in physics, like quantum mechanics, relativity, etc."
Here, L stands for the Lagrangian, which is a measure of energy in a physical system, such as springs, or levers or fundamental particles. "Solving this equation tells you how the system will evolve with time," Cranmer said.
A spinoff of the Lagrangian equation is called Noether's theorem, after the 20th-century German mathematician Emmy Noether. "This theorem is really fundamental to physics and the role of symmetry," Cranmer said. "Informally, the theorem is that if your system has a symmetry, then there is a corresponding conservation law. For example, the idea that the fundamental laws of physics are the same today as tomorrow (time symmetry) implies that energy is conserved. The idea that the laws of physics are the same here as they are in outer space implies that momentum is conserved. Symmetry is perhaps the driving concept in fundamental physics, primarily due to [Noether's] contribution."
Callan-Symanzik Equation
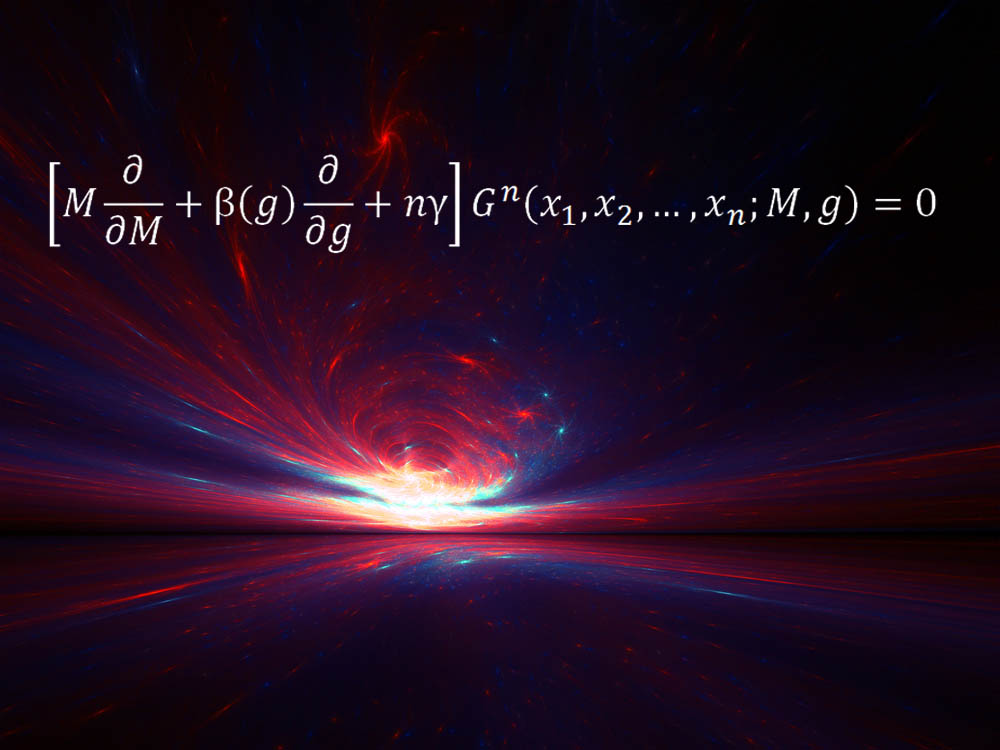
"The Callan-Symanzik equation is a vital first-principles equation from 1970, essential for describing how naive expectations will fail in a quantum world," said theoretical physicist Matt Strassler of Rutgers University.
The equation has numerous applications, including allowing physicists to estimate the mass and size of the proton and neutron, which make up the nuclei of atoms.
Basic physics tells us that the gravitational force, and the electrical force, between two objects is proportional to the inverse of the distance between them squared. On a simple level, the same is true for the strong nuclear force that binds protons and neutrons together to form the nuclei of atoms, and that binds quarks together to form protons and neutrons. However, tiny quantum fluctuations can slightly alter a force's dependence on distance, which has dramatic consequences for the strong nuclear force.
"It prevents this force from decreasing at long distances, and causes it to trap quarks and to combine them to form the protons and neutrons of our world," Strassler said. "What the Callan-Symanzik equation does is relate this dramatic and difficult-to-calculate effect, important when [the distance] is roughly the size of a proton, to more subtle but easier-to-calculate effects that can be measured when [the distance] is much smaller than a proton."
The minimal surface equation
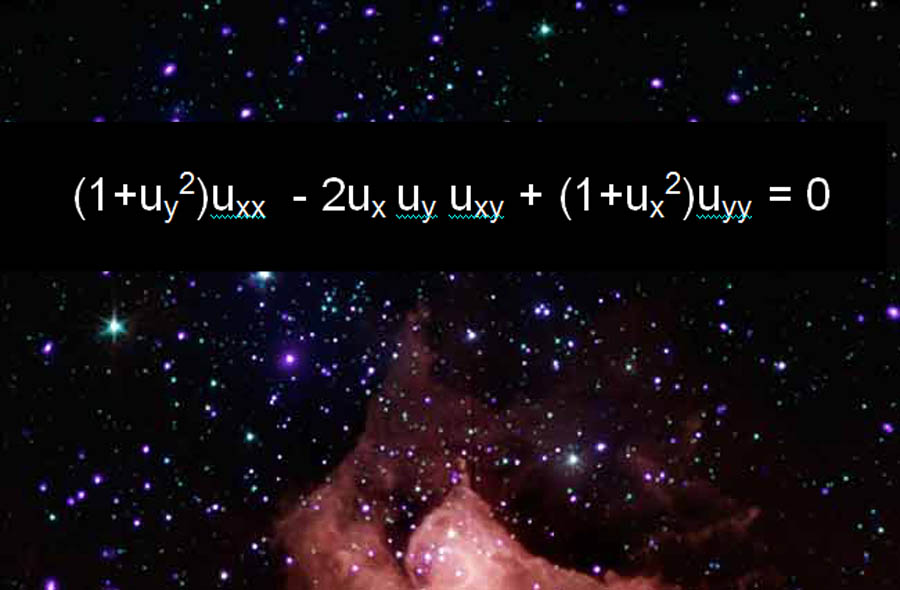
"The minimal surface equation somehow encodes the beautiful soap films that form on wire boundaries when you dip them in soapy water," said mathematician Frank Morgan of Williams College. "The fact that the equation is 'nonlinear,' involving powers and products of derivatives, is the coded mathematical hint for the surprising behavior of soap films. This is in contrast with more familiar linear partial differential equations, such as the heat equation, the wave equation, and the Schrödinger equation of quantum physics."