How long would it take to fall down a hole in the Earth and reach the other side of the planet? Even less time than previously thought, a scientist reveals.
A scenario often presented to introductory physics classes is that of a "gravity tunnel" — a tube drilled from one side of the Earth to the other through the planet's center. The answer taught for nearly a half-century for how long a fall through such a hole would take was about 42 minutes and 12 seconds.
The solution to this problem depends on the strength of Earth's gravitational pull, which in turn is based on its mass. As one falls through the planet, there is less mass beneath whoever is making the descent over time, so the force of the gravity experienced would lessen as one approached Earth's center. [What If? 22 Crazy Hypothetical Questions (and their Answers)]
Still, assuming no air resistance, the momentum from the fall could sling one all the way to the surface on the other side. Anyone making the fall would have to make sure to get away from the exit hole as soon as they flew out of it, or else they could drop in again, hurtling back and forth inside the gravity tunnel, like a weight swinging at the end of a pendulum.
"I guess you can imagine it like a waterslide that takes about 40 minutes to fall through that takes you to speeds over 8 kilometers per second (17,895 mph)," said physicist Alexander Klotz at McGill University in Montreal. "Halfway through the ride, gravity would switch directions and you'd go from right-side up to upside down. You'd have to grab onto the other end or else you'd fall back down the way you came. If the waterslide was made of glass, it would be like zooming through a sea of lava."
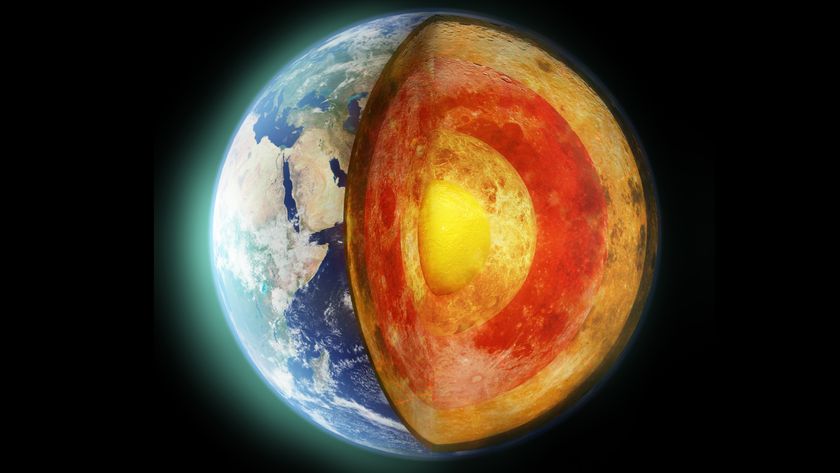
Still, at least one major unrealistic assumption dogged this calculation ever since it was first made in 1966. Ignoring for a moment how drilling a hole about 7,918 miles (12,742 kilometers) long through the Earth is virtually impossible, the problem with the 42-minute solution was that it assumed the planet was uniform in density throughout like a marble.
Now, using a more realistic model of the Earth, Klotz finds the fall would take only about 38 minutes and 11 seconds, about 4 minutes faster than thought.
Klotz based his calculations on the internal structure of the planet as determined from seismic data. While the Earth's crust has a density less than about 187 lbs. per cubic foot (3 grams per cubic centimeter), Earth's center has a density of about 811 lbs. per cubic foot (13 grams per cubic centimeter). The density of the planet does not rise in a straightforward manner the farther down one goes — there is a sharp 50 percent increase in density at the boundary of the planet's mantle and its outer core about 1,800 miles (2,900 km) below Earth's surface.
The physicist assumed there was no air resistance in the gravity tunnel. "In my opinion, if you have the technology to dig such a tunnel, you have the technology to suck the air out," Klotz said.
Surprisingly, Klotz found that he calculated almost the identical answer if he assumed the strength of Earth's gravitational pull was uniform throughout the planet and equal to its value on the surface. This assumption works because Earth's gravity "only changes by, like, 10 percent as you go deeper — first stronger, then weaker — for like the first 3,000 kilometers (1,865 miles)," Klotz said. "So if you start falling and picking up speed, by the time you reach a region in which gravity is significantly different from its surface value, you are going so fast that you spend very little time in this region."
Don't expect anyone to test these calculations with a real tunnel through Earth anytime soon.
"The Soviets tried digging as deep a hole as they could from 1970 to 1989 and only got 12 kilometers (7.5 miles) deep, about 0.1 percent of the way through the Earth," Klotz said.
Klotz detailed his findings in the March issue of the American Journal of Physics.
Follow Live Science @livescience, Facebook & Google+. Original article on Live Science.
